|
See the talk by Shane Ross!
RealVideo of talk: [56k modem] [broadband] [cable/DSL] What is the Interplanetary Superhighway?
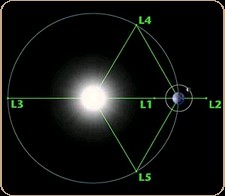
Our solar system is interconnected by a vast system of winding tunnels and conduits in space around the sun and planets which we call the “Interplanetary Superhighway” or IPS for short (Lo and Ross [1], [2]). This ancient and giant labyrinth around the Sun is generated by the Lagrange points of all of the planets and satellites within the solar system. For every three body system (such as the sun-planet-spacecraft system), there are five Lagrange points (also known as libration points). These points are special locations in space where the gravitational forces and the rotational forces within the three body system are balanced, as shown in Figure 1. They were discovered by Euler (L1, L2, L3) and Lagrange (L4, L5).
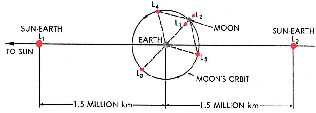
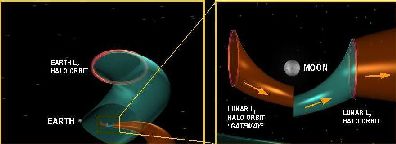
Figure 2 shows schematically the Lagrange points of the Earth-Moon System and their geometric relationship with the Sun-Earth’s L1 and L2. For clarity, we will refer to the lunar Lagrange points as LL1, etc., and the Earth Lagrange Points as EL1, etc. We refer to the region of space around the Earth containing all of these Lagrange points as the “Earth’s neighborhood”. It is a sphere of roughly 1.5 million km (1 million miles) radius around the Earth. Figure 3 provides an artist conception of a portion of the IPS in the Earth’s neighborhood connecting the Lunar LL1 Gateway with spacecraft in orbit about Earth’s EL2 described in [2].
For an exposition on the dynamics of the Lagrange points and the foundations of IPS, see Koon, Lo, Marsden, and Ross [3] and references therein as well as the papers at the recent conference in Spain on “Libration Orbits and Applications” [4]. Brief Historical SketchThe Interplanetary Superhighway is based on a mathematical concept known as invariant manifolds (the tubes). Invariant manifolds are a part of dynamical systems theory (chaos theory) created by Poincaré in his celebrated study of the three body problem in the late nineneenth century. Carles Simó and his group in Barcelona were the first to systematically apply dynamical systems theory to space mission design. Unfortunately, their seminal work for ESA on the SOHO mission was not used. Please see the next two pages for more information on the Interplanetary Superhighway and definitions of technical terms. The work of Simó’s group on invariant manifolds and dynamical systems theory in the late 1980’s gave rise to the question of whether the invariant manifold tubes of one planet might intersect the tubes of another planet to provide a means of transport between the planets? Pursuit of these ideas with many colleagues has resulted in the body of work supporting the theory and use of the Interplanetary Superhighway. When various proposals for libration missions came along, a partnership with Purdue University was formed to develop and apply invariant manifold theory for these missions. This included the design of the Genesis trajectory. Genesis was not the first mission using the pathways within the Interplanetary Superhighway (i.e. the invariant manifolds of the Sun-Earth-Moon system). As it is a natural phenomenon, of course, any object in that vicinity has to use those pathways. However, the theoretical approach and the computation algorithms used to design the Genesis trajectory are based on invariant manifold theory. The fact that the theory of invariant manifolds was used in the design of the Genesis trajectory in no way diminishes the work of Robert Farquhar and others on earlier Lagrange point missions. He not only coined the name “halo orbits”, he convinced NASA to fly the historic first libration mission, ISEE-3/ICE. He opened the door for the rest of the world to follow. Today, libration orbits are not only accepted by the space community, but have become a popular location for a great variety of missions. Many of these have been conceived of by Robert Farquhar. We are building on the work that he and his team began. Definition of the Interplanetary SuperhighwayPlease refer to the technical concepts and definitions section for more details of the terminology used. ·
What is the Interplanetary Superhighway (IPS)? - The Interplanetary Superhighway: The “Interplanetary Superhighway” is a term used to describe the network of tubes formed by low-energy trajectories connecting the bodies of the Solar System. The contribution of this work has been the development of new technologies for space trajectory design and dynamical astronomy using modern mathematical and computational methods in the N-body problem. - Invariant Manifolds of Periodic & Quasiperiodic Orbits: The Interplanetary Superhighway is the network of trajectories generated by the collection of invariant manifolds (stable and unstable manifolds which in the 2 dimensional case form tubes) of all of the unstable periodic and quasiperiodic orbits within the Solar System. - Solar System as Coupled Three Body Problems: The Solar System here is modeled as a series of “coupled circular restricted 3 body problems” (CCRTBP). - Interplanetary Superhighway in Other Solar System Models: For trajectories of short duration such as needed for mission design, the Interplanetary Superhighway can be realized in more sophisticated models of the Solar System (e.g. the JPL ephemeris model) using methods such as multiple shooting (differential correction) or continuation. To study long-term average behavior, symplectic integration may be used. ·
How does it
work? - Provide Interplanetary Transfer Via Intersecting Manifolds (Tubes): Between planets, and between a planet and its satellites, the manifolds (tubes) may intersect in position space (configuration space) to provide opportunities for moving from one manifold to another. - May Require Propulsion: One may be able to travel large distances in the Interplanetary Superhighway without any propulsion. However, this may not always be the case. This is because the manifolds are actually in phase space which includes both position and velocity. Thus for true intersection to occur, the velocities must also match. Since the manifolds more frequently intersect in position space than in phase space, a change in velocity may be required. ·
Why are these
orbits so inexpensive to control? - Trade Time vs. Energy: The energies required by the trajectories to travel on the Interplanetary Superhighway are typically cheaper propulsively than standard transfers. But this may require much longer time. - Chaos Provides Cheap Control: The invariant manifolds of unstable orbits frequently are the sources of chaotic motion. When chaos is present, the orbits become very sensitive. A slight change at one point can cause great variations in the orbital behavior far away. Thus only a small control force is needed to make large changes in the behavior for such trajectories. ·
Distinction
Between Transport vs. Transportation: We make the distinction between the
“Transport of Natural Objects” such as comets and asteroids, and the
“Transportation of Artificial Objects” such as spacecraft. - Transportation Applications are Best in the Planet’s Neighborhood: The Interplanetary Superhighway is most effective in transportation applications in the “neighborhood of a planet”, a large region encompassing the L1 and L2 Lagrange points of the planet. - Impractical for Interplanetary Transfers Due to Long Transfer Time: Due to the long time needed to achieve the low energy transfers between planets, the Interplanetary Superhighway is impractical for transfers such as from Earth to Mars at present. - May Play Significant Role in Low Thrust Trajectory Design and Optimization: Preliminary work indicates invariant manifolds provide the natural low-energy pathways which may be used to speed up the design and optimization of low thrust trajectories. - Applications to the Theory of Transport in the Solar System: The temporary capture of comets by Jupiter such as Gehrels 3, Oterma, Helin-Roman-Crockett have been explained using invariant manifold theory (in the journal of Chaos ) Transport coefficients for asteroids escaping Mars have been computed using invariant manifold theory (as reported in Physical Review Letters) Technical Concepts and DefinitionsIn order to understand the basic components of the Interplanetary Superhighway, some basic concepts and definitions from mathematics are helpful and provided here. · Three Body Problem: The three body problem studies the motions of three bodies moving under the influence of their mutual gravitation such as the motions of the Sun-Earth-Moon system. · Circular Restricted Three Body Problem (CRTBP): The CRTBP is a simplification of the Three Body Problem where the third body is assumed to have very small mass which is infinitesimal. Hence it exerts no force on the two other bodies called Primaries. The motion of a spacecraft under the gravity of the Sun and the Earth can be modeled this way. A further simplification assumes that the primary bodies are moving in circular orbits about one another. This is the CRTBP. · Coupled CRTBP: This is a model of the four body problem such as for a comet moving in the Sun-Jupiter-Saturn system. We model it as two CRTBP’s, the first one is the Sun-Jupiter-Comet CRTBP model, the second is the Sun-Saturn-Comet CRTBP model. Jupiter and Saturn are assumed to not exert gravitational force on one another. We may add additional planets in this fashion to model the solar system as a series of coupled CRTBP’s. ·
Manifold: A
manifold is the mathematical term for a surface of arbitrary dimension.
Familiar 1-dimensional manifolds are
lines, curves in space, the circle, a helix. Familiar 2-dimensional manifolds
are the plane, a sphere, the doughnut (also called torus), and the cylinder. In
our context, the manifold of interest are surfaces made up of trajectories and
orbits. · Periodic Orbit: An orbit which closes on itself. Fixed points, circular, elliptical, and halo orbits are examples of periodic orbits. Topologically, all periodic orbits are either points or deformed circles. · Quasiperiodic Orbit: An orbit which winds around a torus (doughnut-shaped manifold) but which never closes on itself. It is the simplest generalization of the periodic orbit. Lissajous orbits are examples of quasiperiodic orbits. The Genesis orbit around L1 is a Lissajous orbit. · Stable and Unstable Orbit: An orbit is unstable if slight perturbations will cause the spacecraft moving on the orbit to leave the orbit and move far away. Otherwise, it is stable. L1 and L2, halo and Lissajous orbits are all unstable orbits. ·
Invariant
Manifold: An unstable periodic orbit in a Hamiltonian system (a general
class of differential equations which includes the three body problem) have
associated two families of orbits forming tubes connected to the periodic
orbit. One family of orbits asymptotically approaches the periodic orbit. This
family is called the stable manifold.
The other family of orbits asymptotically
departs from the periodic orbit. This family is called the unstable manifold.
Invariant manifold theory was developed by Henri Poincaré in the late nineteenth
century when he studied the three body problem. It is a part of dynamical
systems theory. Unstable quasiperiodic orbits also have similar invariant
manifolds. · Dynamical Systems Theory: Dynamical systems theory is also known as the geometric theory of differential equations or global analysis. It is popularly called “chaos theory”. It was created by Henri Poincare when he studied the three-body problem in the late nineteenth century. Invariant manifold theory is a part of this work. · Deterministic Chaos: This type of chaotic motion is generated not by random statistical fluctuations, but by its extreme sensitive dependence on the initial conditions for the differential equations describing this motion. The effect is a small change at one part of a trajectory can make a great changes elsewhere. This is how low-energy trajectories are obtained. Notes [1] Lo, M., S. Ross, “SURFing the Solar System: Invariant Manifolds and the Dynamics of the Solar System”, JPL IOM 312/97, 1997. [2] M. Lo, S. Ross, “The Lunar L1 Gateway: Portal to the Stars and Beyond”, AIAA Space 2001 Conference, Albuquerque, NM, August 28-30, 2001. [3] W.S. Koon, M. Lo, J. Marsden, S. Ross, “Heteroclinic Orbits between Periodic Orbits and Resonanace Transitions in Celestial Mechanics”, Chaos, Vol. 10, No. 2, June, 2000. [4] The web site of a recent international conference organized with colleagues in Spain on “Libration Orbits and Applications” describes some of the state-of-art work being done by space agencies, universities, and industry around the world using these ideas as well as other methods. It is: http://www.ieec.fcr.es/libpoint/main.html
Back | Home |